40 LAB1.3 Measurement Uncertainty, Accuracy, and Precision
Learning Objectives
By the end of this section, you will be able to:
- Define accuracy and precision
- Distinguish exact and uncertain numbers
- Represent uncertainty in measured and calculated quantities using significant figures
An online mapping program reports that the distance from Portland to Seattle is 174 miles. Is this strictly true? If you make this drive and your trip odometer reports the distance as 180.6 miles, do you think there is something wrong with your measurement?
You probably won’t lose sleep over the difference, for multiple reasons. First, you know that the size of each city produces some uncertainty in that reported distance of 174 miles. Depending on your starting and ending points you could make trips that are actually quite a bit longer or shorter than this – perhaps by 5-10 miles. You also may recognize that when you actually measure the distance with your odometer, the precision of that tool (to 0.1 mi) is greater than the online description. So the tool you use also will affect how you think about the distance measurement.
These sorts of considerations are built into a system used for reporting both measurement (in the car and in the laboratory) and calculations produced with measured numbers. These are collectively referred to as the rules for significant figures.
Significant figures are those digits in an experimentally measured quantity that establish the precision with which the value is known. Note that these rules do not guarantee the accuracy of your measurement. Accuracy is the extent to which a measured value coincides with the true or accepted value. These rules do not guarantee accuracy.
Rather, significant figures rules deal with precision, or the “fineness” (i.e. the number of digits) of the measurement as well as the measurement’s reproducibility. All measuring tools have some degree of uncertainty associated with them. The odometer in your car can not measure down to the inch your car has traveled, so the measurement is only precise to the nearest 0.1 mi. The measurement of mass on a laboratory pan balance also has a limit of precision: typical balances can record masses to the nearest mg or 0.1 mg.
Counting is the only type of measurement that is completely free from uncertainty, provided the number of objects being counted does not change while the counting process is underway. The result of such a counting measurement is an example of an exact number. If we count eggs in a carton, we know exactly how many eggs the carton contains. The numbers of defined quantities are also exact. By definition, 1 foot is exactly 12 inches, 1 inch is exactly 2.54 centimeters, and 1 gram is exactly 0.001 kilogram. Quantities derived from measurements other than counting, however, are uncertain to varying extents due to practical limitations of the measurement process used.
Significant Figures in Measurement
Basics: Making a Measurement
The numbers from measured quantities, unlike defined or directly counted quantities, are not exact. To measure the volume of liquid in a graduated cylinder, you should make a reading at the bottom of the meniscus, the lowest point on the curved surface of the liquid.
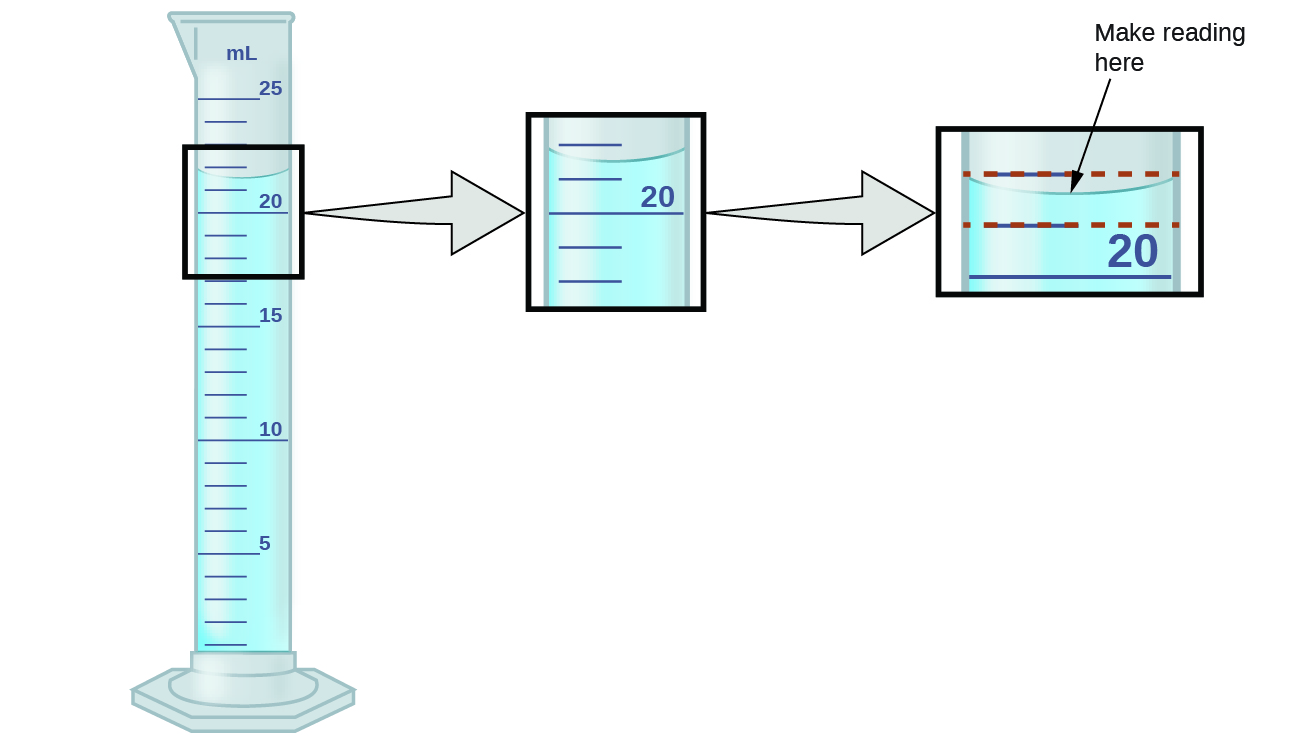
Refer to the illustration in Figure 1. The bottom of the meniscus in this case clearly lies between the 21 and 22 markings, meaning the liquid volume is certainly greater than 21 mL but less than 22 mL. The meniscus appears to be a bit closer to the 22-mL mark than to the 21-mL mark, and so a reasonable estimate of the liquid’s volume would be 21.6 mL. In the number 21.6, then, the digits 2 and 1 are certain, but the 6 is an estimate. Some people might estimate the meniscus position to be equally distant from each of the markings and estimate the tenth-place digit as 5, while others may think it to be even closer to the 22-mL mark and estimate this digit to be 7. Note that it would be pointless to attempt to estimate a digit for the hundredths place, given that the tenths-place digit is uncertain.
This concept holds true for all measurements, even if you do not actively make an estimate. If you place a quarter on a standard electronic balance, you may obtain a reading of 6.72 g. The digits 6 and 7 are certain, and the 2 indicates that the mass of the quarter is likely between 6.71 and 6.73 grams. The quarter weighs about 6.72 grams, with a nominal uncertainty in the measurement of ± 0.01 gram. If we weigh the quarter on a more sensitive balance, we may find that its mass is 6.723 g. This means its mass lies between 6.722 and 6.724 grams, an uncertainty of 0.001 gram.
Every measurement has some uncertainty, which depends on the device used (and the user’s ability). All of the digits in a measurement, including the uncertain last digit, are called significant figures or significant digits. Note that zero may be a measured value; for example, if you stand on a scale that shows weight to the nearest pound and it shows “120,” then the 1 (hundreds), 2 (tens) and 0 (ones) are all significant (measured) values.
Counting Significant Digits
Whenever you make a measurement properly, all the digits in the result are significant. But what if you were analyzing a reported value and trying to determine what is significant and what is not? Well, for starters, all nonzero digits are significant, and it is only zeros that require some thought. We will use the terms “leading,” “trailing,” and “captive” for the zeros and will consider how to deal with them.
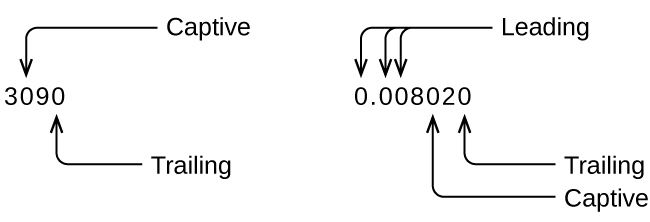
Starting with the first nonzero digit on the left, count this digit and all remaining digits to the right. This is the number of significant figures in the measurement unless the last digit is a trailing zero lying to the left of the decimal point.
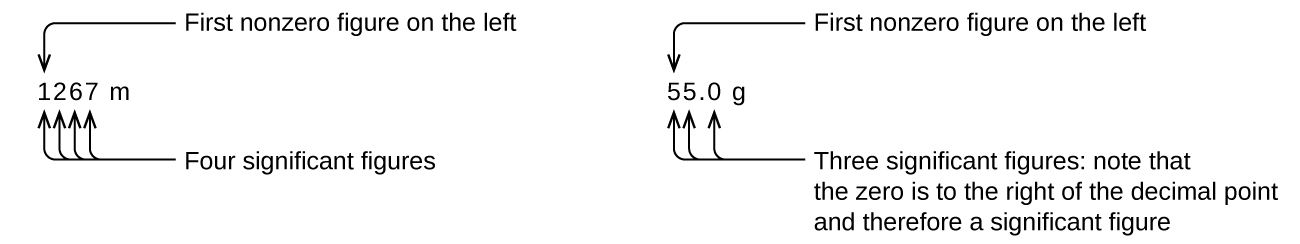
Captive zeros result from measurement and are therefore always significant. Leading zeros, however, are never significant—they merely tell us where the decimal point is located.

The leading zeros in this example are not significant. We could use exponential notation (as described in Appendix B) and express the number as 8.32407 × 10−3; then the number 8.32407 contains all of the significant figures, and 10−3 locates the decimal point.
The number of significant figures is uncertain in a number that ends with a zero to the left of the decimal point location. The zeros in the measurement 1,300 grams could be significant or they could simply indicate where the decimal point is located. The ambiguity can be resolved with the use of exponential notation: 1.3 × 103 (two significant figures), 1.30 × 103 (three significant figures, if the tens place was measured), or 1.300 × 103 (four significant figures, if the ones place was also measured). In cases where only the normal notation is used (1300 g), all trailing zeros are not significant, therefore the measurement would have two significant figures. Though, if the measurement was express with an explicit decimal place (1300. g), then the trailing zeros would be significant, and therefore the measurement would have four significant figures.
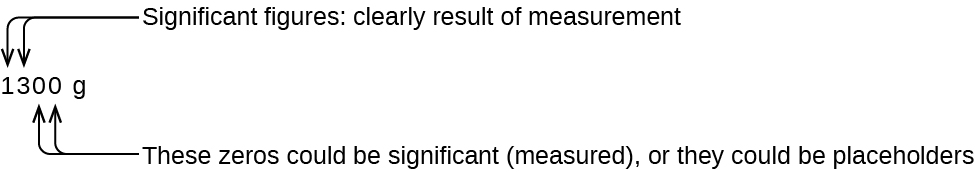

Example 1
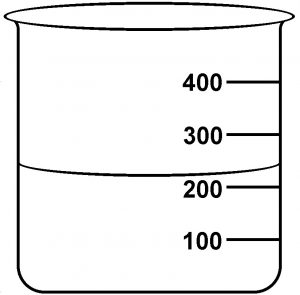
Determine the correct measurement reading for the following volume:
Solution
Because the beaker has gradations of 100 mL, we know that it is at least 200 mL. It is the tens position that is uncertain. Thus we can only record to that position. The reading should be 220 mL. Better still—clarify the significant figures by using scientific notation, which would be 2.2 x 102 mL.
Test Yourself
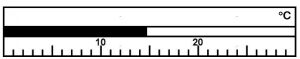
Determine the correct reading for the following temperature:
Answer
14.8oC
Example 2
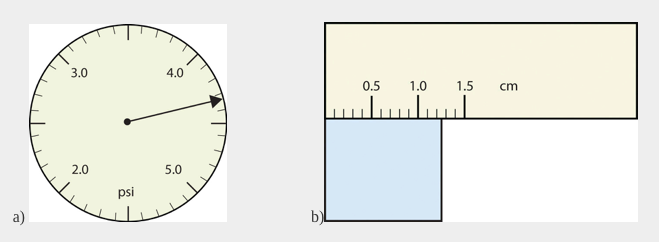
Use each diagram to report a measurement to the proper number of significant figures.
Solution
a) The arrow is between 4.0 and 5.0, so the measurement is at least 4.0. The arrow is between the third and fourth small tick marks, so it’s at least 0.3. We will have to estimate the last place. It looks like about one-third of the way across the space, so let us estimate the hundredths place as 3. Combining the digits, we have a measurement of 4.33 psi (psi stands for “pounds per square inch” and is a unit of pressure, like air in a tire). We say that the measurement is reported to three significant figures.
b)The rectangle is at least 1.0 cm wide but certainly not 2.0 cm wide, so the first significant digit is 1. The rectangle’s width is past the second tick mark but not the third; if each tick mark represents 0.1, then the rectangle is at least 0.2 in the next significant digit. We have to estimate the next place because there are no markings to guide us. It appears to be about halfway between 0.2 and 0.3, so we will estimate the next place to be a 5. Thus, the measured width of the rectangle is 1.25 cm. Again, the measurement is reported to three significant figures.
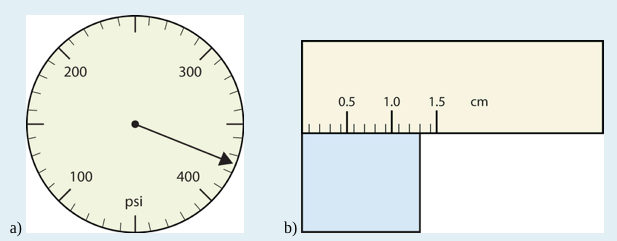
Test Yourself
What would be the reported width of this rectangle?
Answer
0.63 cm
Example 3
Give the number of significant figures in each measurement.
a) 36.7 m b) 0.006606 s c) 2,002 kg d) 306,490,000 people
Solution
a) By rule 1, all nonzero digits are significant, so this measurement has three significant figures.
b) By rule 4, the first three zeros are not significant, but by rule 2 the zero between the sixes is; therefore, this number has four significant figures.
c) By rule 2, the two zeros between the twos are significant, so this measurement has four significant figures.
d) The four trailing zeros in the number are not significant, but the other five numbers are, so this number has five significant figures.
Test Yourself
Give the number of significant figures in each measurement.
a) 0.000601 m b) 65.080 kg
Answers
a) three significant figures b) five significant figures
Example 4
Determine the number of significant figures in the following measurements
a) 0.002040 g b) 300 mL c) 3.021 x 105 g d) 31 pencils (counted exactly, not an estimate)
Solution
a) 4 significant figures. The leading zeros do not count, but the trailing zero does (as there’s a decimal point).
b) By the above rules, 1 significant figure, because there’s no decimal point showing; 300. mL would be 3 sig figs. (To emphasize 1 sig fig it would be better to write the measurement in scientific notation, as 3 x 102 mL, rather than just “300 mL”.)
c) 4 significant figures. (No leading or trailing zeros.) Note that we only consider the digits in the numerical portion of the scientific notation, not the power of 10.
d) Unlimited significant figures. This is an exact number, as it has been counted.
Test Yourself
Determine the number of significant figures in the following measurements.
a) 0.00100 m b) 2.0900 x 103 mL c) 100.0 oC
Answers
a) 3 significant figures b) 5 significant figures c) 4 significant figures
When determining significant figures, be sure to pay attention to reported values and think about the measurement and significant figures in terms of what is reasonable or likely when evaluating whether the value makes sense.
Significant Figures in Calculations
Imagine that drive to Seattle, as described above, took three and a half hours. You could determine your average speed on the trip by taking the distance (in miles) and dividing it by the time required (in hours). Using my calculator to determine 174 ÷ 3.5 I get this:
49.71428571
Since the number here represents miles divided by hours, I could use the unit label mi/hour or “miles per hour.” Note that “per” here translates to “for each,” in terms of its meaning. But look at all those digits! This does not seem reasonable. How many of these digits are meaningful?
A second important principle of uncertainty is that results calculated from a measurement can’t be more precise than the measurement itself. We must take the uncertainty in our measurements into account to avoid misrepresenting the uncertainty in calculated results.
One way to do this is to report the result of a calculation with the correct number of significant figures, which is determined by the following three rules for rounding numbers:
- When we add or subtract numbers, we should round the result to the same number of decimal places as the number with the least number of decimal places (the least precise value in terms of addition and subtraction).
- When we multiply or divide numbers, we should round the result to the same number of digits as the number with the least number of significant figures (the least precise value in terms of multiplication and division).
- If the digit to be dropped (the one immediately to the right of the digit to be retained) is less than 5, we “round down” and leave the retained digit unchanged; if it is more than 5, we “round up” and increase the retained digit by 1.
The following examples illustrate the application of this rule in rounding a few different numbers to three significant figures:
- 0.028675 rounds “up” to 0.0287 (the dropped digit, 7, is greater than 5)
- 18.3384 rounds “down” to 18.3 (the dropped digit, 3, is less than 5)
- 6.8752 rounds “up” to 6.88 (the dropped digit is 5)
Let’s work through these rules with a few examples.
Example 5
Round the following to the indicated number of significant figures:
a) 31.57 (to two significant figures)
b) 8.1649 (to three significant figures)
Solution
a) 31.57 rounds “up” to 32 (the dropped digit is 5)
b) 8.1649 rounds “down” to 8.16 (the dropped digit, 4, is less than 5)
Test Yourself
Round the following to the indicated number of significant figures:
a) 0.424 (to two significant figures)
b) 0.0038661 (to three significant figures)
Answers
a) 0.42 b) 0.00387
Example 6
Rule: When we add or subtract numbers, we should round the result to the same number of decimal places as the number with the least number of decimal places (i.e., the least precise value in terms of addition and subtraction).
Perform the following calculations taking significant figures into account.
a) Add 1.0023 g and 4.383 g.
b) Subtract 421.23 g from 486 g.
Solution
a) Answer is 5.385 g (round to the thousandths place; three decimal places)
b) Answer is 65 g (round to the ones place; no decimal places)

Test Yourself
a) Add 2.334 mL and 0.31 mL.
b) Subtract 55.8752 m from 56.533 m.
Answers
a) 2.64 mL b) 0.658 m
Example 7
Express the final answer to the proper number of significant figures.
a) 101.2 + 18.702 = ?
b) 202.88 − 1.013 = ?
Solution
a) If we use a calculator to add these two numbers, we would get 119.902. However, most calculators do not understand significant figures, and we need to limit the final answer to the tenths place. Thus, we drop the 02 and report a final answer of 119.9 (rounding down).
b) A calculator would answer 201.867. However, we have to limit our final answer to the hundredths place. Because the first number being dropped is 7, which is greater than 7, we round up and report a final answer of 201.87.
Test Yourself
Express the answer for 3.445 + 90.83 − 72.4 to the proper number of significant figures.
Answer
21.9
Example 8
Express the final answer to the proper number of significant figures.
a) 76.4 × 180.4 = ?
b) 934.9 ÷ 0.00455 = ?
Solution
a) The first number has three significant figures, while the second number has four significant figures. Therefore, we limit our final answer to three significant figures: 76.4 × 180.4 = 13,782.56 = 13,800.
b) The first number has four significant figures, while the second number has three significant figures. Therefore we limit our final answer to three significant figures: 934.9 ÷ 0.00455 = 205,472.5275… = 205,000.
Test Yourself
Express the final answer to the proper number of significant figures.
a) 22.4 × 8.314 = ?
b) 1.381 ÷ 6.02 = ?
Answers
a) 186 b) 0.229
In the midst of all these technicalities, it is important to keep in mind the reason why we use significant figures and rounding rules—to correctly represent the certainty of the values we report and to ensure that a calculated result is not represented as being more certain than the least certain value used in the calculation.

Need a refresher or more practice with significant figures? Visit this site (https://viuvideos.viu.ca/media/Significant+Figures/0_t8xwe4s9) to go over the basics of significant figures.
Video source: Significant figures by keyj
Example 9
Experimental Determination of Density Using Water Displacement
A piece of rebar is weighed and then submerged in a graduated cylinder partially filled with water, with results as shown.
a) Use these values to determine the density of this piece of rebar.
b) Rebar is mostly iron. Does your result in (a) support this statement? How?
Solution
The volume of the piece of rebar is equal to the volume of the water displaced:
(rounded to the nearest 0.1 mL, per the rule for addition and subtraction)
The density is the mass-to-volume ratio:
(rounded to two significant figures, per the rule for multiplication and division)
From Table 3 in Chapter 2.2 Measurements, the density of iron is 7.9 g/cm3, very close to that of rebar, which lends some support to the fact that rebar is mostly iron.
Test Yourself
An irregularly shaped piece of a shiny yellowish material is weighed and then submerged in a graduated cylinder, with results as shown.

a) Use these values to determine the density of this material.
b) Do you have any reasonable guesses as to the identity of this material? Explain your reasoning.
Answers
a) 19 g/cm3; (b) It is likely gold; the right appearance for gold and very close to the density given for gold in Table 3 in Chapter 2.2 Measurements.
Accuracy and Precision
Scientists typically make repeated measurements of a quantity to ensure the quality of their findings and to know both the precision and the accuracy of their results. Measurements are said to be precise if they yield very similar results when repeated in the same manner. A measurement is considered accurate if it yields a result that is very close to the true or accepted value. Precise values agree with each other; accurate values agree with a true value. These characterizations can be extended to other contexts, such as the results of an archery competition (Figure 3).
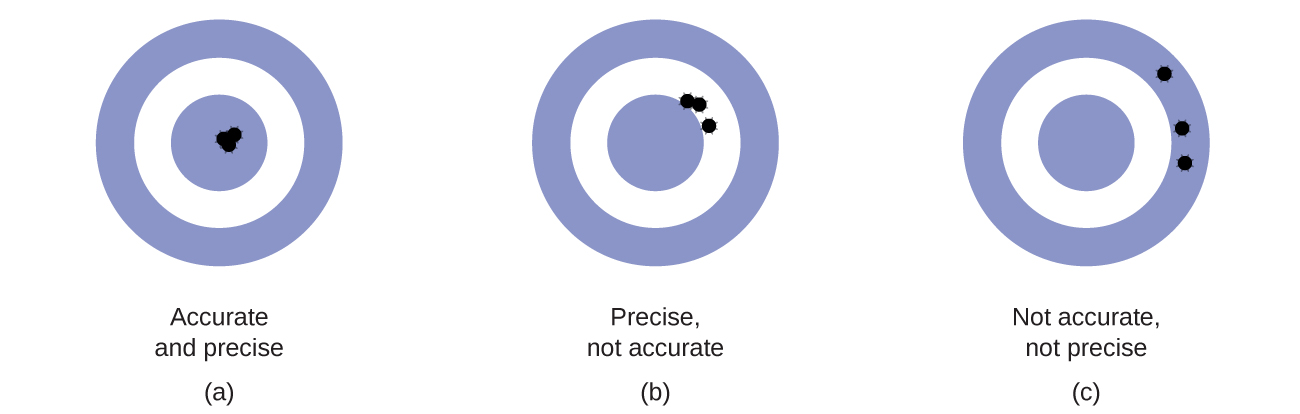
Suppose a quality control chemist at a pharmaceutical company is tasked with checking the accuracy and precision of three different machines that are meant to dispense 10 ounces (296 mL) of cough syrup into storage bottles. She proceeds to use each machine to fill five bottles and then carefully determines the actual volume dispensed, obtaining the results tabulated in Table 1.
| Dispenser #1 | Dispenser #2 | Dispenser #3 |
|---|---|---|
| 283.3 | 298.3 | 296.1 |
| 284.1 | 294.2 | 295.9 |
| 283.9 | 296.0 | 296.1 |
| 284.0 | 297.8 | 296.0 |
| 284.1 | 293.9 | 296.1 |
| Table 1. Volume (mL) of Cough Medicine Delivered by 10-oz (296 mL) Dispensers | ||
Considering these results, she will report that dispenser #1 is precise (values all close to one another, within a few tenths of a milliliter) but not accurate (none of the values are close to the target value of 296 mL, each being more than 10 mL too low). Results for dispenser #2 represent improved accuracy (each volume is less than 3 mL away from 296 mL) but worse precision (volumes vary by more than 4 mL). Finally, she can report that dispenser #3 is working well, dispensing cough syrup both accurately (all volumes within 0.1 mL of the target volume) and precisely (volumes differing from each other by no more than 0.2 mL).
Key Concepts and Summary
Quantities can be exact or measured. Measured quantities have an associated uncertainty that is represented by the number of significant figures in the measurement. The uncertainty of a calculated value depends on the uncertainties in the values used in the calculation and is reflected in how the value is rounded. Measured values can be accurate (close to the true value) and/or precise (showing little variation when measured repeatedly).
Review-Reflect, Extend
2. How many significant figures do these numbers have?
a) 23 b) 23.0 c) 0.00023 d) 0.0002302
3. How many significant figures do these numbers have?
a) 5.44 × 108 b) 1.008 × 10−5 c) 43.09 d) 0.0000001381
4. Compute and express each answer with the proper number of significant figures, rounding as necessary.
a) 56.0 + 3.44 = ? b) 0.00665 + 1.004 = ?
5. Compute and express each answer with the proper number of significant figures, rounding as necessary.
a) 56.7 × 66.99 = ? b) 1.000 ÷ 77 = ?
6. Write the number 87,449 in scientific notation with four significant figures.
7. Write the number 0.000066600 in scientific notation with five significant figures.
8. Perform each calculation and limit each answer to three significant figures.
a) 67,883 × 0.004321 = ?
b) (9.67 × 103) × 0.0055087 = ?
9. Indicate whether each of the following can be determined exactly or must be measured with some degree of uncertainty:
a) the number of seconds in an hour
b) the number of pages in this book
c) the number of grams in your weight
d) the number of grams in 3 kilograms
e) the volume of water you drink in one day
f) the distance from San Francisco to Kansas City
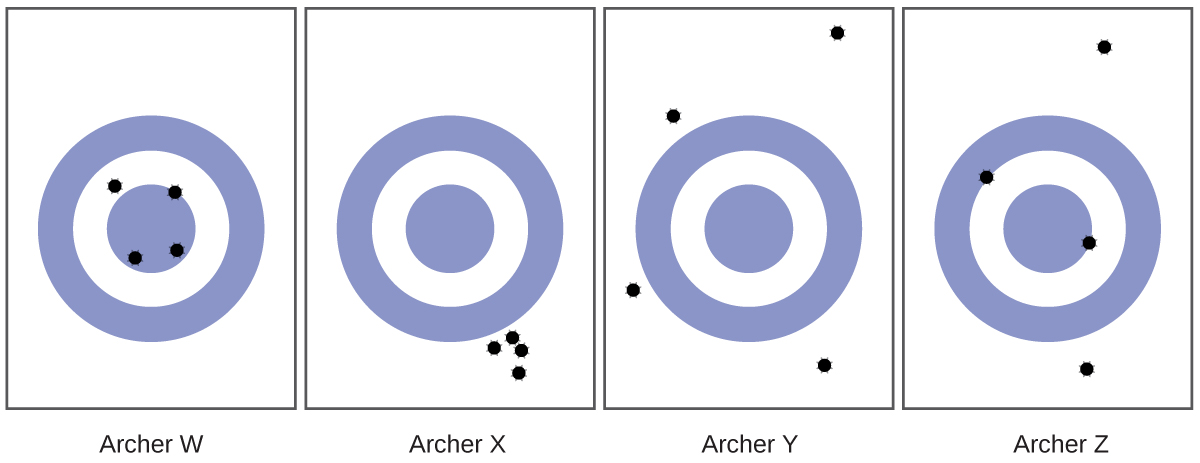
10. Consider the results of the archery contest shown in this figure.
a) Which archer is most precise?
b) Which archer is most accurate?
c) Who is both least precise and least accurate?
Answers
1. a) 375 psi b) 1.30 cm
2. a) two b) three c) two d) four
3. a) three b) four c) four d) four
4. a) 59.4 b) 1.011
5. a) 3.80 × 103 b) 0.013
6. 8.745 × 104
7. 6.6600 x 10−5
8. a) 293 b) 53.3
9. a) exact b) exact c) uncertain d) exact e) uncertain f) uncertain
10. a) Archer X b) Archer W c) Archer Y
Glossary
accuracy: how closely a measurement aligns with a correct value
exact number: number derived by counting or by definition
precision: how closely a measurement matches the same measurement when repeated
rounding: procedure used to ensure that calculated results properly reflect the uncertainty in the measurements used in the calculation
significant figures: (also, significant digits) all of the measured digits in a determination, including the uncertain last digit
uncertainty: estimate of amount by which measurement differs from true value