6.1 Identifying Chiral Carbons

Introduction: Louis Pasteur and the discovery of molecular chirality

In 1848, a 25 year old chemist named Louis Pasteur made a startling – and some thought brash – claim to the scientific community. Pasteur was inexperienced, to say the least: he had only earned his doctorate the previous year, and had just started his first job as an assistant to a professor at the Ecole normale superieure, a university in Paris. Jean-Baptiste Biot, a highly respected physicist who had already made major contributions to scientific fields as diverse as meteorites, magnetism, and optics, was intrigued but unconvinced by Pasteur’s claim. He invited the young man to come to his laboratory and reproduce his experiments.

Decades earlier, Biot had discovered that aqueous solutions of some biologically-derived substances, such as tartaric acid, quinine, morphine, and various sugars, were optically active: that is, the plane of polarized light would rotate in either a positive (clockwise, or right-handed) or negative (counter-clockwise, or left-handed) direction when passed through the solutions. Nobody understood the source of this optical property. One of the biological substances known to be optically active was a salt of tartaric acid, a compound found in abundance in grapes and a major by-product of the wine-making industry.

The compound was dextrorotatory in solution – in other words, it rotated plane-polarized light in the positive (right-handed, or clockwise) direction. Curiously, though, chemists had also found that another form of processed tartaric acid was optically inactive, despite that fact that it appeared to be identical to the optically active acid in every other respect. The optically inactive compound was called ‘acide racemique‘, from the Latin racemus, meaning ‘bunch of grapes’.

Louis Pasteur’s claims had to do with experiments he said he had done with the ‘racemic’ acid. Jean-Babtise Biot summoned Pasteur to his laboratory, and presented him with a sample of racemic acid which he himself had already confirmed was optically inactive. With Biot watching over his shoulder, and using Biot’s reagents, Pasteur prepared the salt form of the acid, dissolved it in water, and left the aqueous solution in an uncovered flask to allow crystals to slowly form as the water evaporated.

Biot again summoned Pasteur to the lab a few days later when the crystallization was complete. Pasteur placed the crystals under a microscope, and began to painstakingly examine their shape, just as he had done in his original experiments. He had recognized that the crystals, which had a regular shape, were asymmetric: in other words, they could not be superimposed on their mirror image. Scientists referred to asymmetric crystals and other asymmetric objects as being ‘chiral’, from the Greek word for ‘hand’. Your hands are chiral objects, because although your right hand and your left hand are mirror images of one another, they cannot be superimposed. That is why you cannot fit your right hand in a left-handed glove.

More importantly, Pasteur had claimed that the chiral crystals he was seeing under the lens of his microscope were of two different types, and the two types were mirror images of each other: about half were what he termed ‘right handed’ and half were ‘left-handed’. He carefully separated the right and left-handed crystals from each other, and presented the two samples to Biot. The eminent scientist then took what Pasteur told him were the left-handed crystals, dissolved them in water, and put the aqueous solution in a polarimeter, an instrument that measures optical rotation. Biot knew that the processed tartaric acid he had provided Pasteur had been optically inactive. He also knew that unprocessed tartaric acid from grapes had right-handed optical activity, whereas left-handed tartaric acid was unheard of. Before his eyes, however, he now saw that the solution was rotating light to the left. He turned to his young colleague and exclaimed, ” Mon cher enfant, j’ai tant aime ́ les sciences dans ma vie que cela me fait battre le coeur!’ (My dear child, I have loved science so much during my life that this makes my heart pound!)

Biot had good reason to be so profoundly excited. Pasteur had just conclusively demonstrated, for the first time, the concept of molecular chirality: molecules themselves – not just macroscopic objects like crystals – could exhibit chirality, and could be separated into distinct right-handed and left-handed ‘stereoisomers’. Tying together ideas from physics, chemistry, and biology, he had shown that nature could be chiral at the molecular level, and in doing do he had introduced to the world a new subfield which came to be known as ‘stereochemistry’.

About ten years after his demonstration of molecular chirality, Pasteur went on to make another observation with profound implications for biological chemistry. It was already well known that ‘natural’ tartaric acid (the right-handed kind from grapes) could be fermented by bacteria. Pasteur discovered that the bacteria were selective with regard to the chirality of tartaric acid: no fermentation occurred when the bacteria were provided with pure left-handed acid, and when provided with racemic acid they specifically fermented the right-handed component, leaving the left-handed acid behind.

Pasteur was not aware, at the time of the discoveries described here, the details of the structural features of tartaric acid at the molecular level that made the acid chiral, although he made some predictions concerning the bonding patterns of carbon which turned out to be remarkably accurate. In the more than 150 years since Pasteur’s initial tartaric acid work, we have greatly expanded our understanding of molecular chirality. Put simply, stereochemistry is the study of how bonds are oriented in three-dimensional space. It is difficult to overstate the importance of stereochemistry in nature, and in the fields of biology and medicine in particular. As Pasteur so convincingly demonstrated, life itself is chiral: living things recognize different stereoisomers of organic compounds and process them accordingly.

So what, structurally, is a chiral object? The term chiral, from the Greek work for ‘hand’, refers to anything which exhibits ‘handedness.’ Your hands, of course, are the origin of this term as the prototypical chiral objects. But so are most gloves – you cannot fit your left hand into a right-handed glove, so gloves can come in right-handed and left-handed versions.

If you examine the origin of this handedness in any familiar object that is chiral, you’ll find that the relationship between right and left-handed chiral objects are that they are mirror images of one another, and not superimposable.

Consider the example of a pair of garden gloves. If you place the right-handed glove over the top of the left-handed glove with the palm surfaces both facing downward, the thumbs point in opposite directions. If you orient the two gloves so the thumbs and fingers overlay one another, then the palms are facing opposite directions.

Also, if you hold the right-handed glove up before a mirror and imagine superimposing the glove with the glove’s image in the mirror, the fingers will be aligned (all could lay over one another, thumb to thumb, etc.) but the palm surfaces will be on opposite sides. The image in the mirror is actually identical to a left-handed glove!

This same phenomenon occurs in any ‘handed’ object you interact with, and as Pasteur discovered, it also characterizes some objects at the molecular scale.

Another way to consider the phenomenon of chirality is to note that the gloves do not have an internal mirror plane of symmetry: you cannot find any way to slice straight through the glove in such a way that one piece of the glove is a mirror image of the other side. The ‘slice’ defines what is called a ‘mirror plane,’ which is a plane (2D flat surface). The symmetry we are searching for occurs when the two sides of the plane (two pieces of the glove) are mirror images of one another. Chiral objects do not have such a plane of symmetry.

A pair of pants, on the other hand is achiral – lacking chirality – because, if you ignore some details, you could slice through the pants and have two halves that are mirror images of one another. Where is that plane? It bisects the pants from the crotch through the waist, with one leg and half of the seat of the pants on each side. This is the internal mirror plane of symmetry. You don’t need to buy pants in “right handed” or “left handed” versions, and the mirror image of a pair of pants could be superimposed over the originals.

For Pasteur, Biot, and their contemporaries the source of chirality at the molecular level was at first undeciphered. It stood to reason that a chiral molecule is one that does not contain a plane of symmetry, and thus cannot be superimposed on its mirror image.

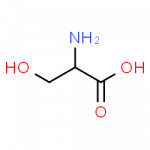
We now know much more about chemical structure. For molecules to have no internal mirror plane of symmetry, to not be superimposable on their mirror images, we know they must contain one or more chiral centers. These are almost always tetrahedral carbons with four different substituent groups around them. Consider the cartoon molecule A below: here we have four different substituents denoted by balls of four different colors around a carbon:

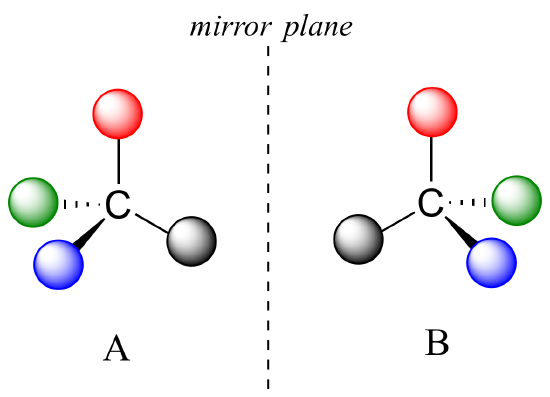
The mirror image of A, which we will call B, is drawn on the right side of the figure. Notice that every point on A lines up with the same point on B: in other words, if you looked at A in the mirror, you would see B as the image.

If we could simply slide structure A to see if it is superimposable with B, laying the carbon on A over the top of the carbon on B, we would also be able to align the black and red balls but not the blue and green ones. The blue sphere on A (left) would overlap the green sphere on B, and the green sphere on A would overlap the blue sphere on B.

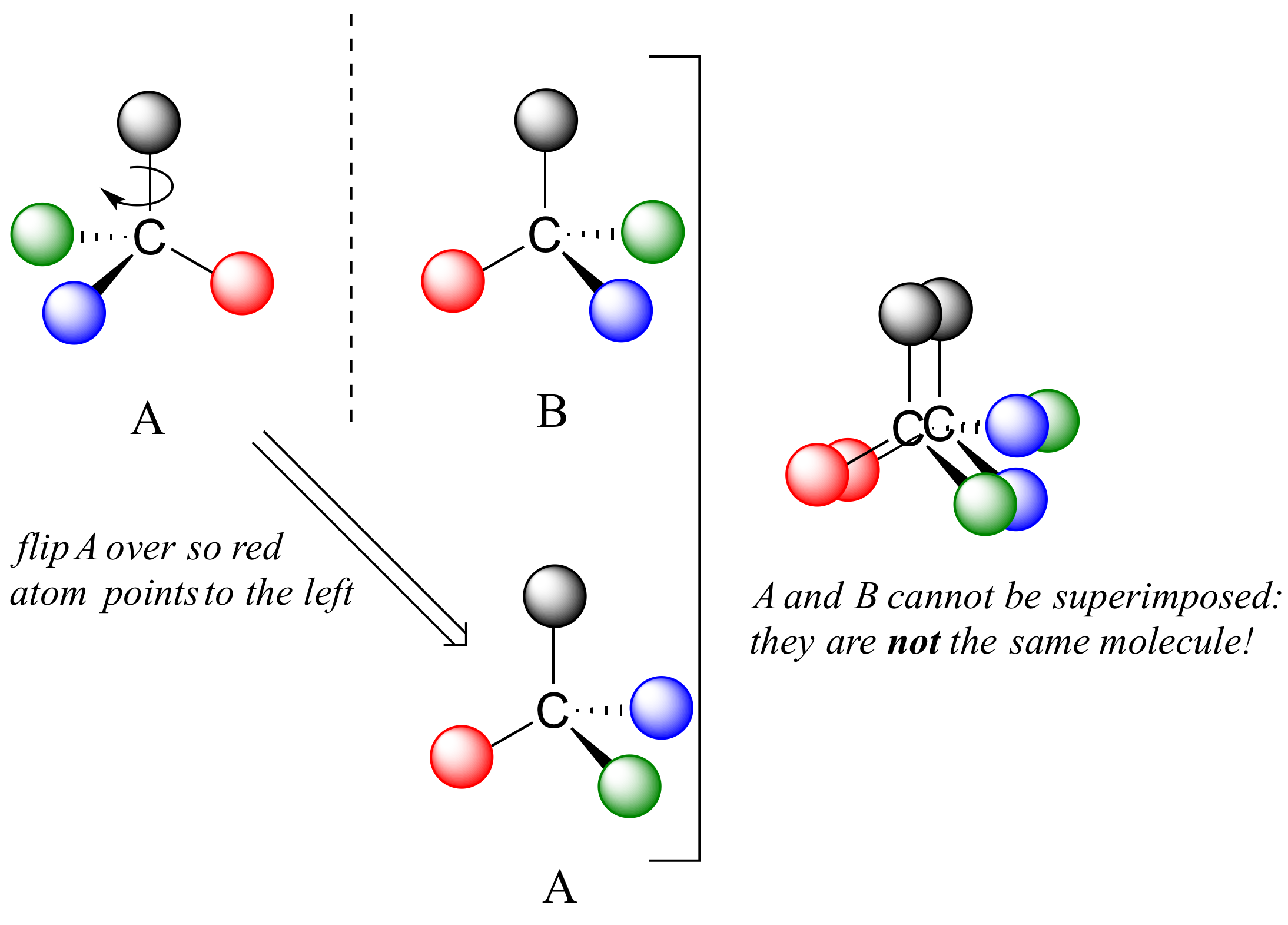
A is not superimposable on its mirror image (B), thus by definition A is a chiral molecule. It follows that B also is not superimposable on its mirror image (A), and thus it is also a chiral molecule.

Notice also that neither A nor B has an internal plane of symmetry. There is no way to slice through these molecules so that the two portions are mirror images of one another.

The relationship between molecules A and B is that of stereoisomers: molecules which share a molecular formula and also same connectivity to other groups, but which have a different arrangement of atoms in three-dimensional space. There are two types of stereoisomers: enantiomers and diastereomers. Enantiomers are pairs of stereoisomers which are mirror images of each other: this describes molecules A and B. A chiral molecule will always have one and only one enantiomer: the word enantiomer describes one member of a pair of molecules, and the term implies a relationship to the other compound.

Enantiomers have identical physical properties (melting point, boiling point, density, and so on). However, enantiomers do differ in how they interact with polarized light and they may also interact in very different ways with other chiral molecules – proteins, for example. They are different chemical substances, though similar in many ways.

The other type of stereochemical relationship is that of a diastereomer. Diastereomers are stereoisomers which are not mirror images of each other. These can occur under certain circumstances, such as when there are multiple chiral centers arranged in specific ways on a molecule. Diastereomers share molecular formulas and connectivity between atoms, but their physical properties differ from one another.

We defined a chiral center as a tetrahedral carbon with four different substituents. If, instead, a tetrahedral carbon has two identical substituents (two black atoms in the cartoon figure below), then while it still has a mirror image (everything has a mirror image, unless we are talking about a vampire!), it is superimposable on its mirror image.

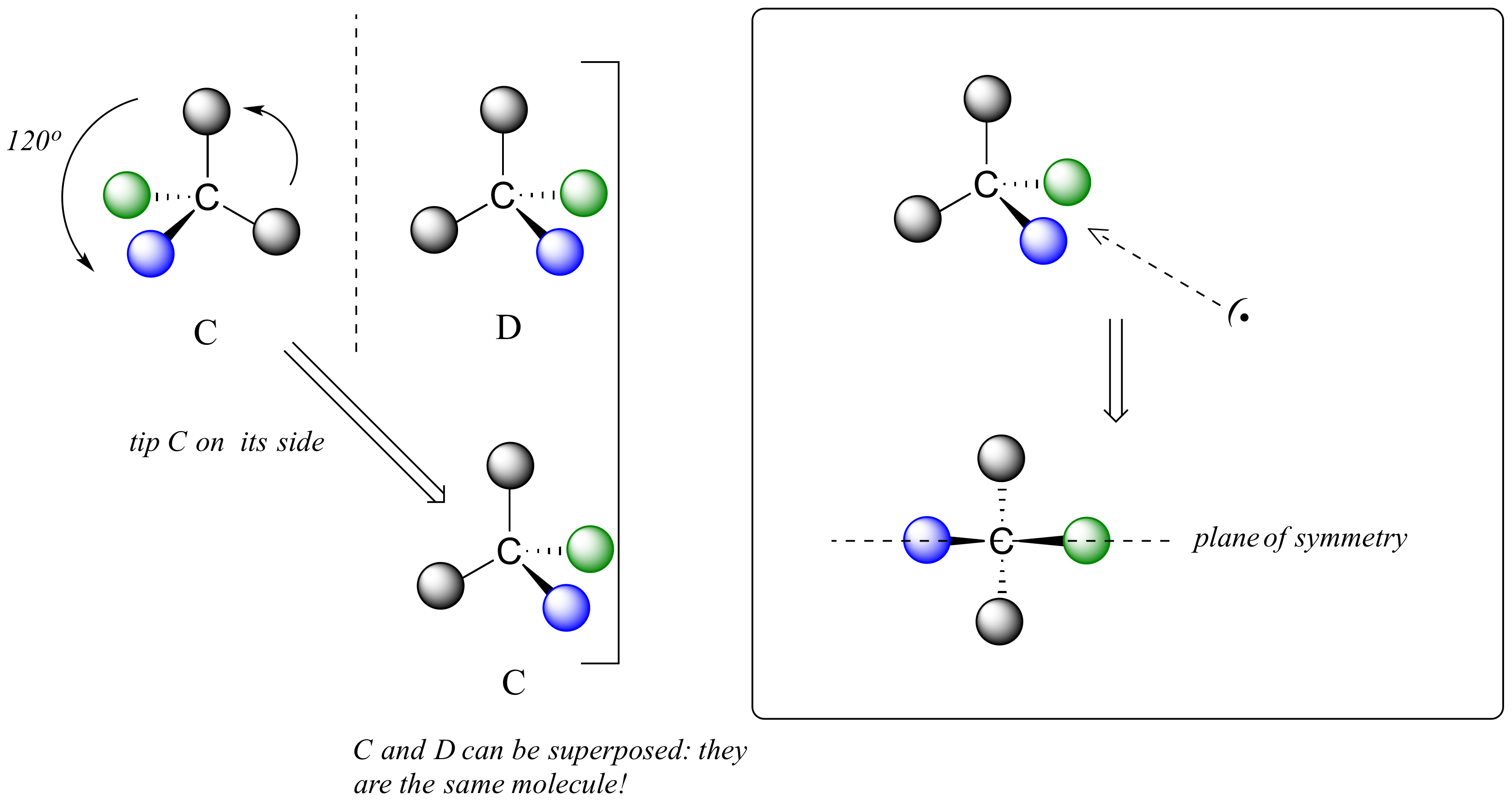
The molecule has an internal plane of symmetry that passes through the central carbon and the green and blue spheres. Imagine slicing the molecule in half so that these 3 atoms are bisected: the two pieces would be mirror images of one another.

For these reasons we know the molecule is achiral. Using the same reasoning, we can see that a carbon with only three groups around it is also not a chiral center.

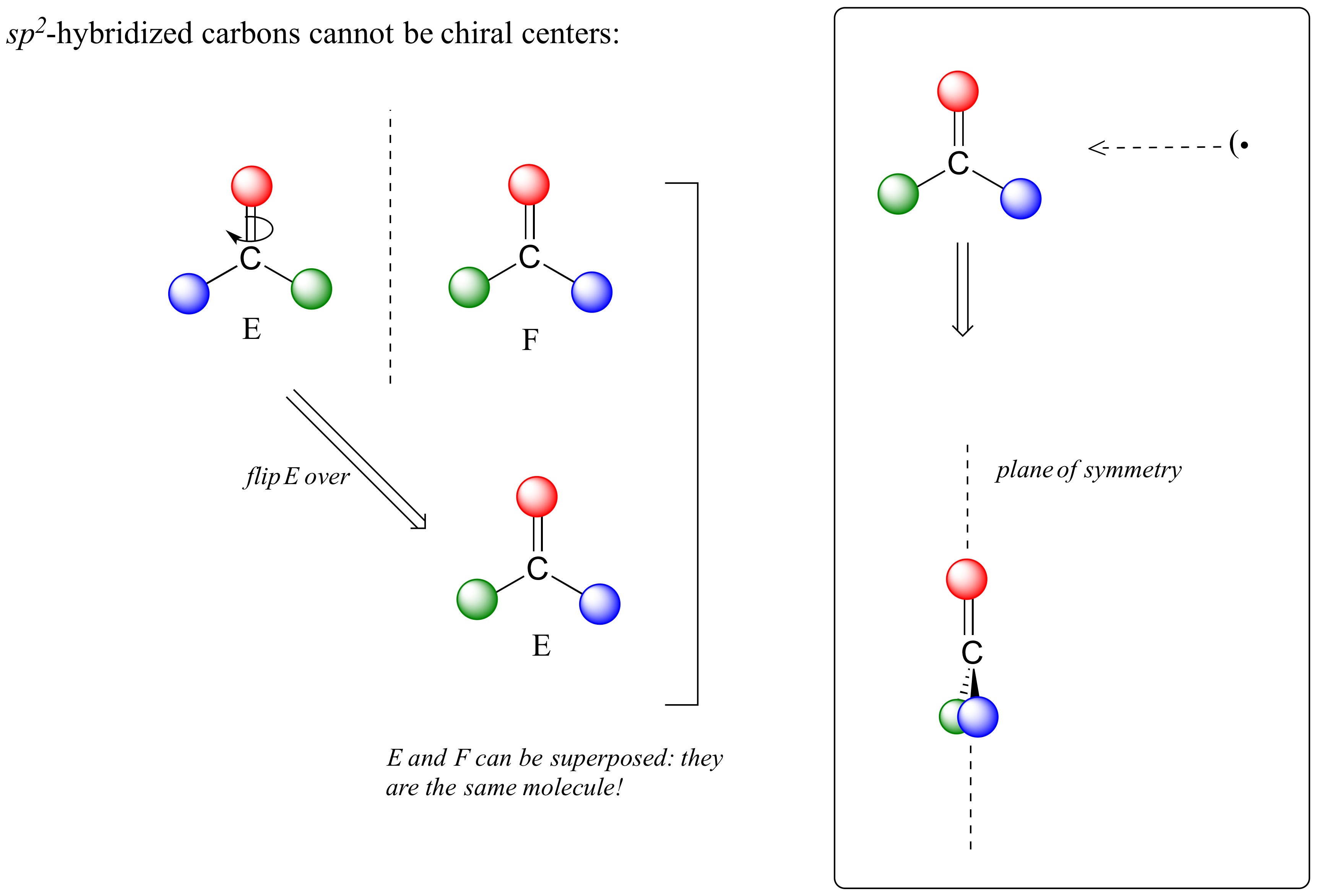
Notice that structure E can be superimposed on F, its mirror image – all you have to do is pick E up, flip it over, and it can completely overlay F. This molecule has an internal plane of symmetry, and is achiral.

Let’s apply our general discussion to real molecules. For now, we will limit our discussion to molecules with a single chiral center. It turns out that tartaric acid, the subject of our chapter introduction, has two chiral centers, making the situation with that molecule a bit more complex.

Consider 2-butanol, drawn in two dimensions below.

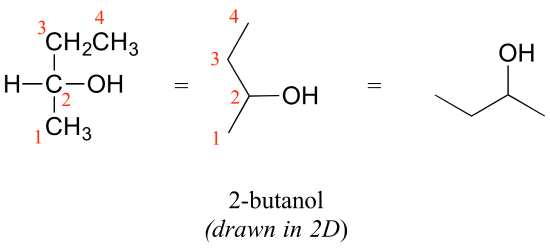
Carbon #2 is a chiral center: it has four bonds to other atoms and is tetrahedral, and each of the four things attached to it is different: a hydrogen, a methyl (-CH₃) group, an ethyl (-CH₂CH₃) group, and a hydroxyl (OH) group. If we put carbon 2 in the center of a 3-dimensional drawing and lay out the 4 groups around it, we can investigate the stereochemistry. To start we can connect the OH and ethyl groups with regular lines, and show the attachment to the methyl group with a solid wedge and the attachment to hydrogen with a dashed wedge. A mirror image of our first structure, with all the same groups, can also be drawn.

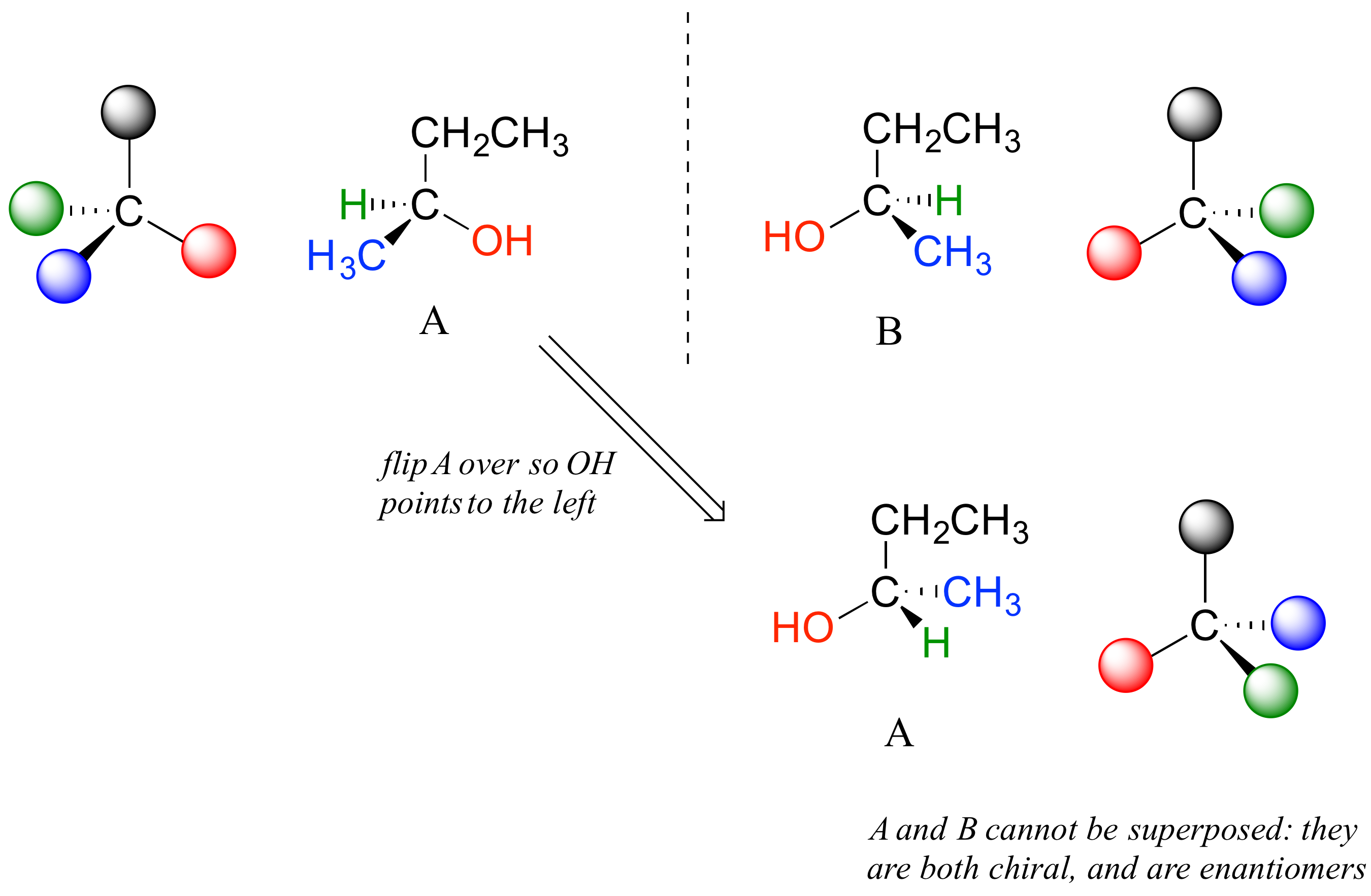
When we try to superimpose A onto B, we find that we cannot do it. A and B are both chiral molecules, and they are enantiomers of each other.

The substance 2-propanol, unlike 2-butanol, is not a chiral molecule. Carbon #2 is bonded to two identical substituents (methyl groups), not 4 different groups, and so it is not a chiral center. It has an internal mirror plane. Its mirror image is superimposable over the original.

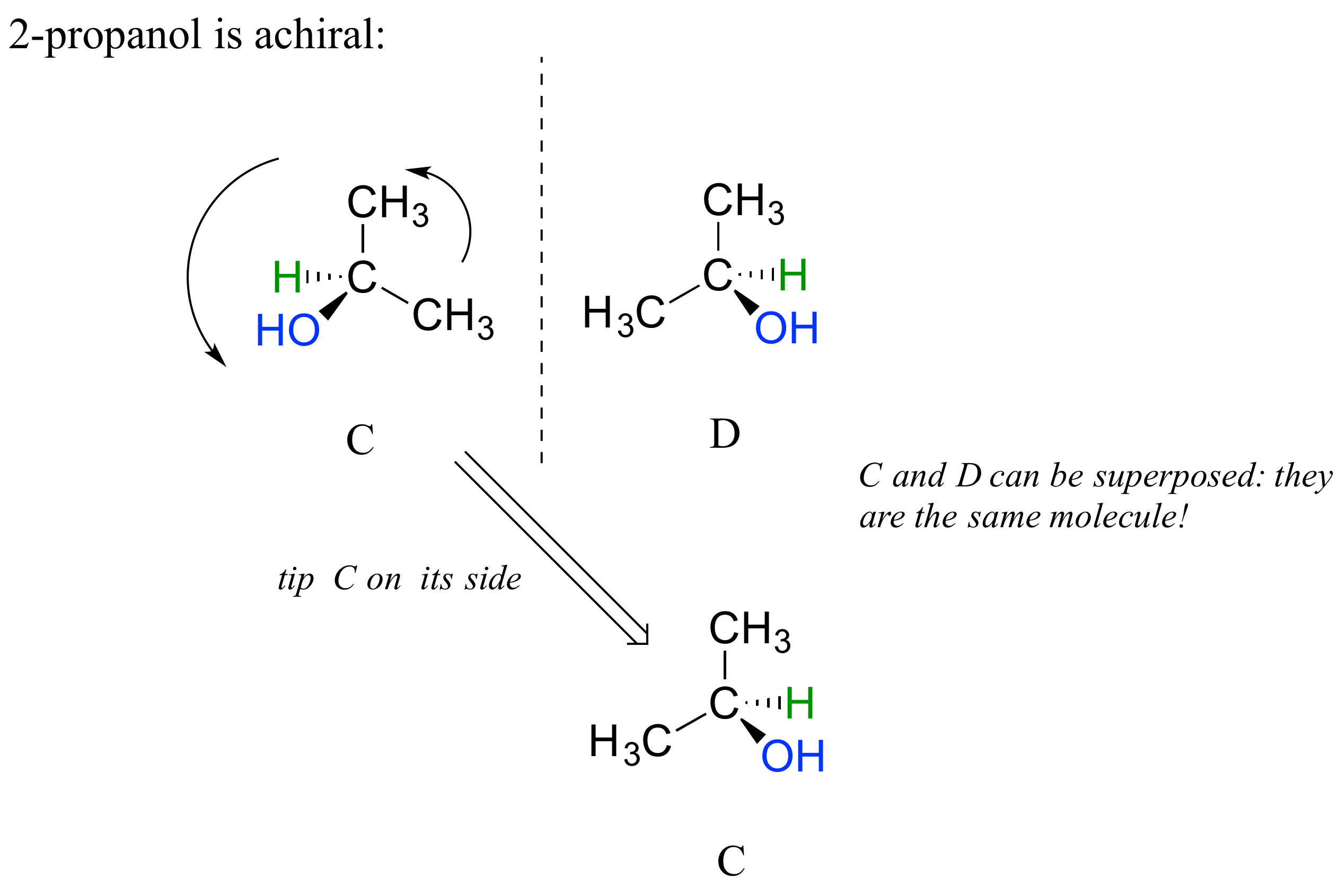
Notice that 2-propanol is superimposable on its own mirror image. Structures C and D here show two views of the same compound.

When we look at very simple molecules like 2-butanol, it is not too difficult to draw out the mirror image and recognize that it is not superimposable. However, with larger, more complex molecules, this can be a daunting challenge in terms of drawing and three-dimensional visualization. The easy way to determine if a molecule is chiral is simply to look for the presence of one or more chiral centers: molecules with chiral centers will often be chiral. We can not say ‘always’ here because it is possible to come up with exceptions to this rule.

Here’s another trick to make your stereochemical life easier: if you want to draw the enantiomer of a chiral molecule, it is not necessary to go to the trouble of drawing the point-for-point mirror image, as we have done up to now for purposes of illustration. Instead, keep the carbon skeleton the same, and simply reverse the solid and dashed wedge bonds on the chiral carbon: that accomplishes the same thing. You should use models to convince yourself that this is true, and also to convince yourself that swapping any two substituents about the chiral carbon will result in the formation of the enantiomer.

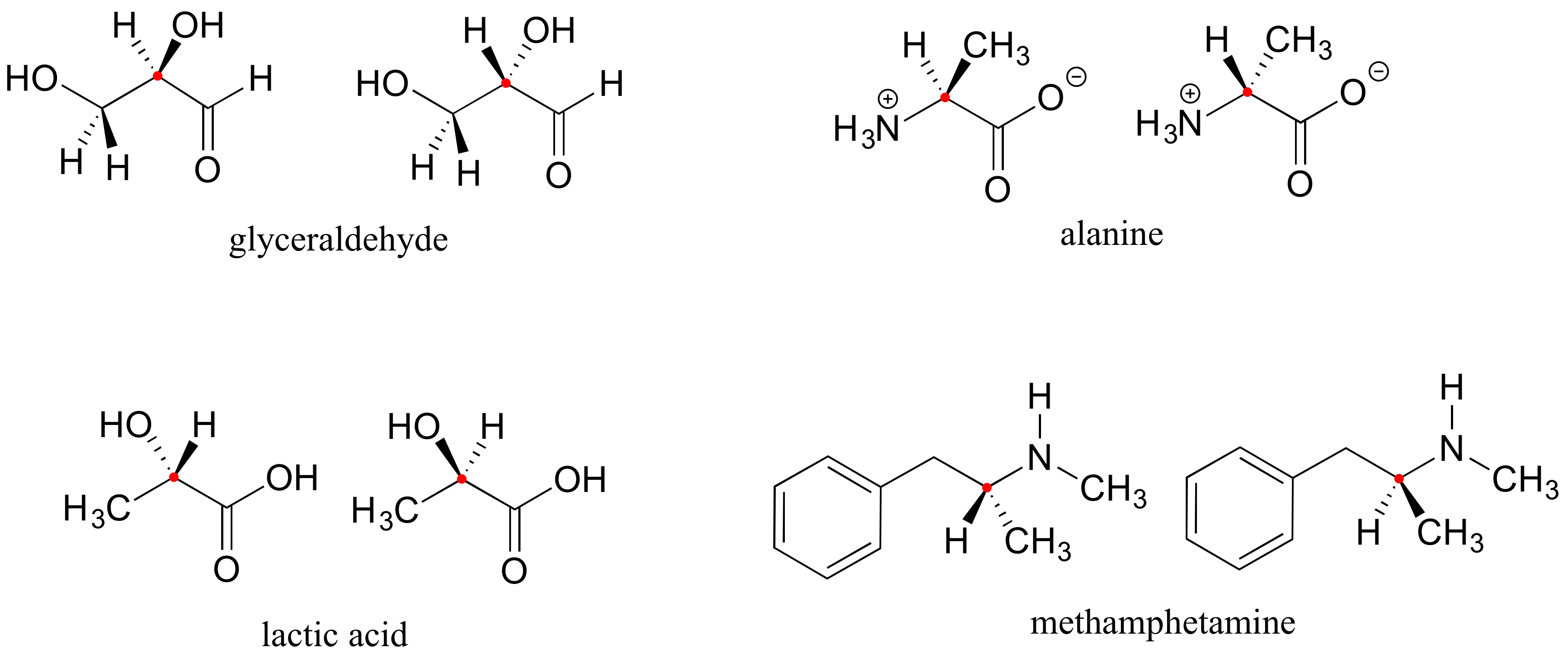
Here are four more examples of chiral biomolecules, each one shown as a pair of enantiomers, with chiral centers marked by red dots.

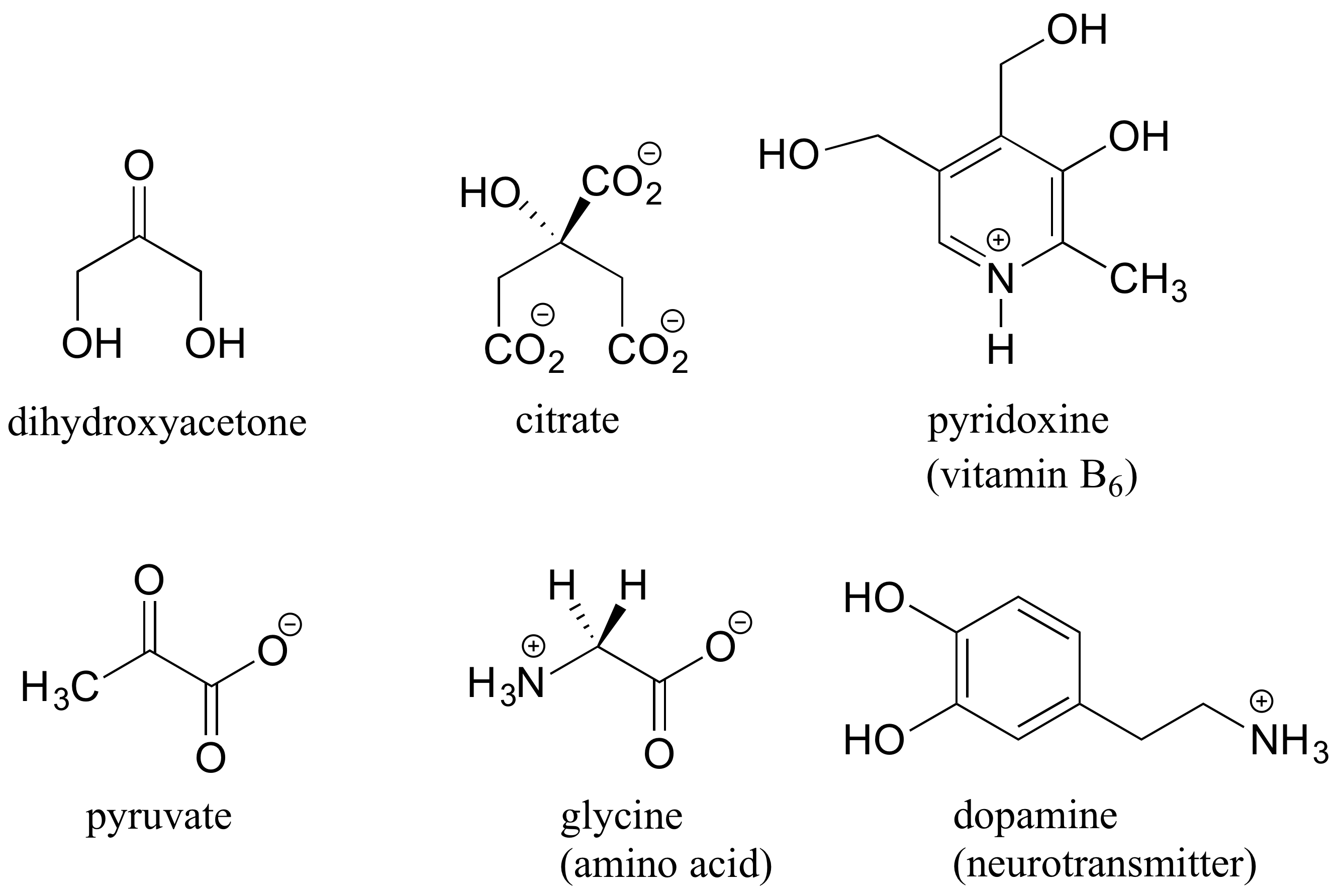
Here are some examples of achiral biomolecules – convince yourself that none of them contain a chiral center:

Can a chiral center be something other than a tetrahedral carbon with four different substituents? The answer to this question is ‘yes’ – however, these alternative chiral centers are very rare in the context of biological organic chemistry, and outside the scope of our discussion here.