6 Precision and GPE

When someone is selling a used car, its mileage might be listed as 80,000 miles or 82,000 miles because a buyer will want to know the approximate mileage but doesn’t need an exact value. If you buy the car, however, you’ll need to know the mileage to the nearest mile when you’re completing the registration paperwork.
Precision
The precision of a number is the place value of the rightmost significant figure. For example, ![]() is precise to the thousands place,
is precise to the thousands place, ![]() is precise to the ones place, and something like
is precise to the ones place, and something like ![]() would be precise to the tenths place.
would be precise to the tenths place.
Exercises
Identify the precision (i.e., the place value of the rightmost significant figure) of each number.
Precision-Based Rounding
In Module 3, we used precision-based rounding because we were rounding to a specified place value; for example, rounding to the nearest tenth. Let’s practice this with overbars and trailing zeros.
Precision-based rounding:
-
Locate the rounding digit in the place to which you are rounding.
-
Look at the test digit directly to the right of the rounding digit.
-
If the test digit is 5 or greater, increase the rounding digit by 1 and drop all digits to its right. If the test digit is less than 5, keep the rounding digit the same and drop all digits to its right.
Remember, when the rounding digit of a whole number is a ![]() that gets rounded up to a
that gets rounded up to a ![]() , we must write an overbar above that
, we must write an overbar above that ![]() .
.
Also, when the rounding digit of a decimal number is a ![]() that gets rounded up to a
that gets rounded up to a ![]() , we must include the
, we must include the ![]() in that decimal place.
in that decimal place.
Exercises
Round each number to the indicated place value. Be sure to include an overbar or trailing zeros if necessary.
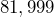 (thousands)
(thousands)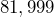 (hundreds)
(hundreds)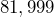 (tens)
(tens)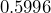 (tenths)
(tenths)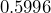 (hundredths)
(hundredths)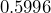 (thousandths)
(thousandths)
Precision when Adding and Subtracting
Suppose the attendance at a large event is estimated at ![]() people, but then you see
people, but then you see ![]() people leave. Is the new estimate
people leave. Is the new estimate ![]() ? No, because the original estimate was precise only to the nearest thousand. We can’t start with an imprecise number and finish with a more precise number. If we estimated that
? No, because the original estimate was precise only to the nearest thousand. We can’t start with an imprecise number and finish with a more precise number. If we estimated that ![]() people had left, then we could revise our attendance estimate to
people had left, then we could revise our attendance estimate to ![]() because this estimate maintains the same level of precision as our original estimate.
because this estimate maintains the same level of precision as our original estimate.
Don’t round off the original numbers; do the necessary calculations first, then round the answer as your last step.
Exercises
Add or subtract as indicated. Round to the appropriate level of precision.
- Find the combined weight of four packages with the following weights:
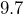 lb,
lb,  lb,
lb, 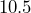 lb,
lb, 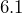 lb.
lb. - Find the combined weight of four packages with the following weights:
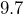 lb,
lb,  lb,
lb, 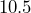 lb,
lb, 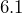 lb.
lb. - While purchasing renter’s insurance, Chandra estimates the value of her insurable possessions at
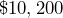 . After selling some items valued at
. After selling some items valued at 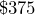 , what would be the revised estimate?
, what would be the revised estimate? - Chandra knows that she has roughly
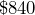 in her checking account. After using her debit card to make two purchases of
in her checking account. After using her debit card to make two purchases of 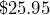 and
and 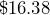 , how much would she have left in her account?
, how much would she have left in her account?
 Before we move on, let’s circle back to multiplication and division again. If you are multiplying by an exact number, you can consider this a repeated addition. For example, suppose you measure the weight of an object to be
Before we move on, let’s circle back to multiplication and division again. If you are multiplying by an exact number, you can consider this a repeated addition. For example, suppose you measure the weight of an object to be ![]() ounces and you want to know the weight of three of these objects; multiplying
ounces and you want to know the weight of three of these objects; multiplying ![]() times
times ![]() is the same as adding
is the same as adding ![]() ounces. The answer is still precise to the hundredths place. When working with an exact number, we can assume that it has infinitely many significant figures. (Treat exact numbers like royalty; their accuracy is perfect and it would be an insult to even question it.)
ounces. The answer is still precise to the hundredths place. When working with an exact number, we can assume that it has infinitely many significant figures. (Treat exact numbers like royalty; their accuracy is perfect and it would be an insult to even question it.)
Greatest Possible Measurement Error (GPE)
Suppose you are weighing a dog with a scale that displays the weight rounded to the nearest pound. If the scale says Sir Barks-A-Lot weighs ![]() pounds, he could weigh anywhere from
pounds, he could weigh anywhere from ![]() pounds to almost
pounds to almost ![]() pounds. The true weight could be as much as
pounds. The true weight could be as much as ![]() pounds above or below the measured weight, which we could write as
pounds above or below the measured weight, which we could write as ![]() .
.
Now suppose you are weighing Sir Barks-A-Lot with a scale that displays the weight rounded to the nearest tenth of a pound. If the scale says Sir Barks-A-Lot weighs ![]() pounds, we now know that he could weigh anywhere from
pounds, we now know that he could weigh anywhere from ![]() pounds to almost
pounds to almost ![]() pounds. The true weight could be as much as
pounds. The true weight could be as much as ![]() pounds above or below the measured weight, which we could write as
pounds above or below the measured weight, which we could write as ![]() .
.
As we increase the level of precision in our measurement, we decrease the greatest possible measurement error or GPE. The GPE is always one half the precision; if the precision is to the nearest tenth, ![]() , the GPE is half of one tenth, or five hundredths,
, the GPE is half of one tenth, or five hundredths, ![]() . The GPE will always be a
. The GPE will always be a ![]() in the place to the right of the place value of the number’s precision.
in the place to the right of the place value of the number’s precision.
Another way to think about the GPE is that it gives the range of values that would round off to the number in question. ![]() tells us a lower value and an upper value.
tells us a lower value and an upper value. ![]() is the lowest weight that would round up to
is the lowest weight that would round up to ![]() , and
, and ![]() is the upper limit of the weights that would round down to
is the upper limit of the weights that would round down to ![]() . (Yes, 23.5 technically would round up to 24, but it is easier to just say
. (Yes, 23.5 technically would round up to 24, but it is easier to just say ![]() instead of
instead of ![]() .) Using inequalities, we could represent
.) Using inequalities, we could represent ![]() as the range of values
as the range of values ![]() .
.
Exercises

The attendance at a Portland Thorns match is estimated to be ![]() people.
people.
- What is the precision of this estimate?
- What is the greatest possible error in this estimate?
A roll of plastic sheeting is ![]() inches thick.
inches thick.
- What is the precision of this measurement?
- What is the greatest possible error in this measurement?
Plastic sheeting ![]() inches thick is referred to as
inches thick is referred to as ![]() mil.
mil.
- What is the precision of this measurement, in mils?
- What is the greatest possible measurement error, in mils?
Summary of Accuracy, Precision, GPE
Here is a summary of the important terms from Modules 5 & 6. It is easy to get them mixed up, but remembering that “precision” and “place value” both start with “p” can be helpful.
Summary of Terms
Significant figures: the digits in a number that we trust to be correct
Accuracy: the number of significant figures
When multiplying or dividing, we use the accuracy to round the result.
Precision: the place value of the rightmost significant digit
Greatest possible measurement error (GPE): one half the precision
When adding or subtracting, we use the precision to round the result.
Exercises
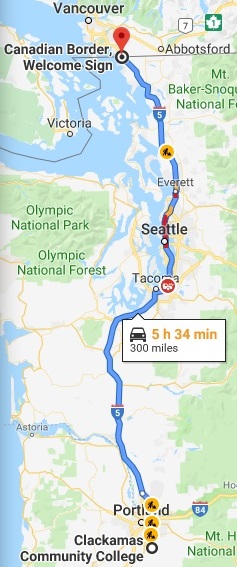
Google Maps says that the driving distance from CCC’s main campus to the Canadian border, rounded to the nearest mile, is ![]() miles.
miles.
- Write the distance with an overbar showing the correct precision.
- What is the precision?
- What is the GPE?
Google Maps says that the driving time, rounded to the nearest minute, is ![]() hours and
hours and ![]() minutes.
minutes.
- What is the precision of this estimate?
- What is the GPE of this estimate?
Of course, the traffic conditions will change during the trip, making the estimate of ![]() hours and
hours and ![]() minutes unrealistically precise. Let’s assume that the drive will take 5.5 hours.
minutes unrealistically precise. Let’s assume that the drive will take 5.5 hours.
- What is the accuracy of this estimate?
- What is the accuracy of the distance?
- Calculate the average speed of the vehicle, rounded appropriately.
- Why did we need to consider the accuracy instead of the precision for this calculation?

