33 Non-Right Triangle Trigonometry

You may use a calculator throughout this module.
Basic trigonometry applies to right triangles, but there are two useful formulas that can be used with non-right triangles; these are known as the Law of Sines and the Law of Cosines.
The Law of Sines allows you to use a proportion to solve for a missing value in a triangle.
The Law of Cosines is essentially the Pythagorean Theorem with an extra twist that makes it work with any kind of triangle.
These formulas have applications in many fields, most obviously in surveying and forestry where distances cannot be measured directly.
Law of Sines
Suppose we have a triangle with its vertices labeled A, B, C, and the sides opposite each vertex labeled a, b, and c.
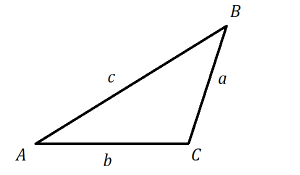
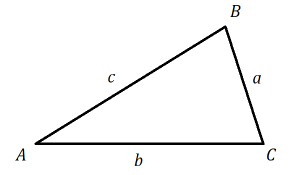
The Law of Sines is useful when you are dealing with two sides and two angles; if you know three of those values, you can use the formula to figure out the fourth value. Depending on which sides and angles you know, you’ll use one of these three versions:
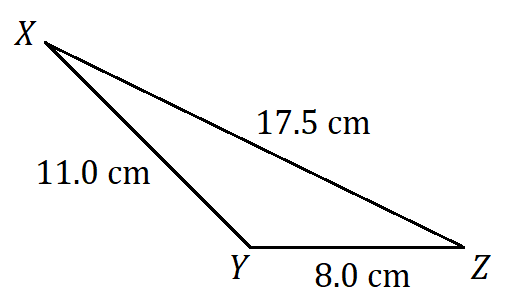
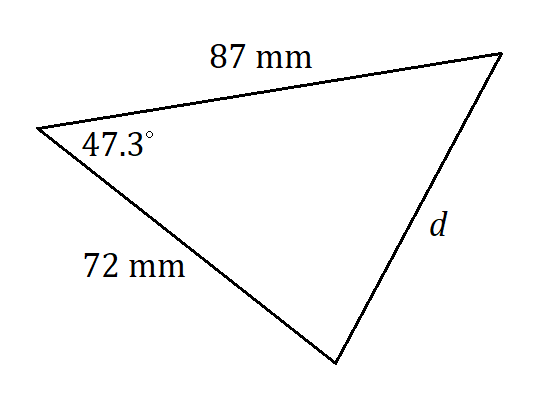
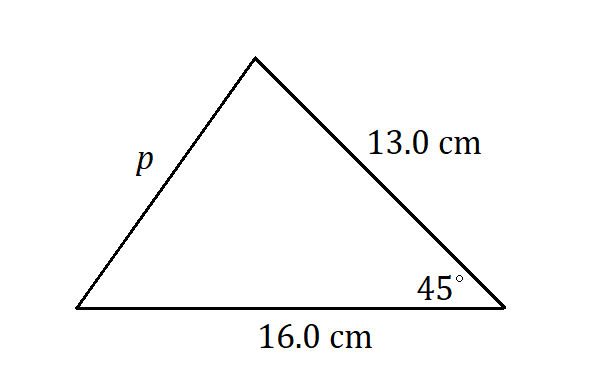
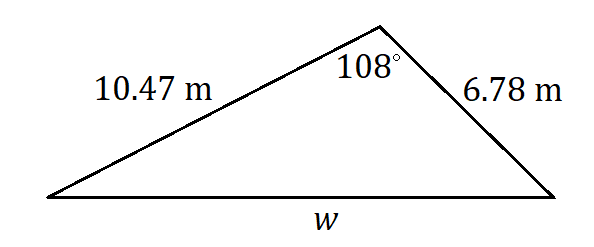
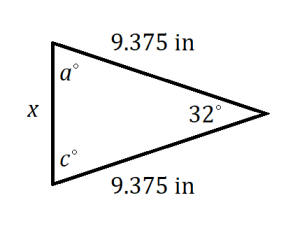
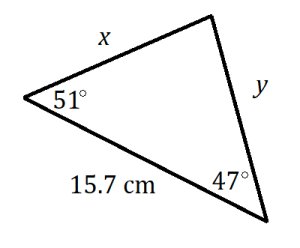
Exercises
Determine the unknown value(s) in each triangle.

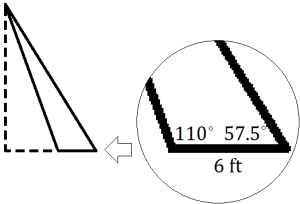
- A utility pole is supported by two guy wires as shown in the figure below. The shorter wire makes a
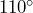 angle with the sidewalk, the longer wire makes a
angle with the sidewalk, the longer wire makes a  angle with the sidewalk, and the wires meet the sidewalk a distance of 6 feet from each other. Determine the approximate length of each wire.
angle with the sidewalk, and the wires meet the sidewalk a distance of 6 feet from each other. Determine the approximate length of each wire.
When we need to determine an angle measure, we sometimes have a situation with two possible results: the angle could be acute or obtuse. (For this ambiguous situation to arise, we must know the lengths of two sides and the measure of an acute angle that is not between those two sides.) The inverse sine function on a calculator will always be programmed to give an acute angle measure for the result. If it is clear that the angle should be obtuse, simply subtract the calculator’s result from 180 degrees.
Exercises
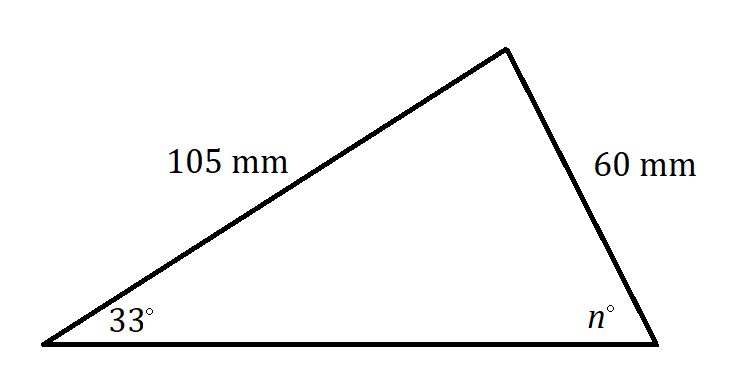
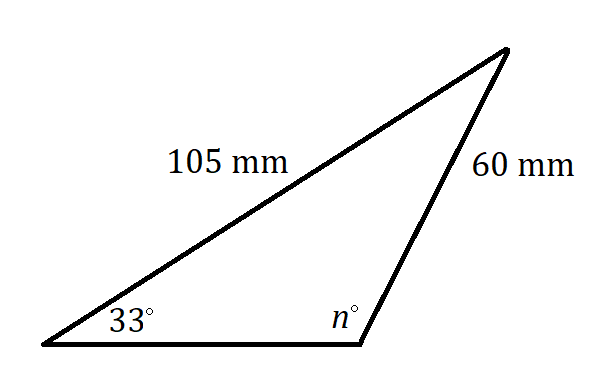
Determine the unknown angle measure in each triangle.
- Assume that n represents an acute angle.
- Assume that n represents an obtuse angle.
Law of Cosines
Again, suppose we have a triangle with its vertices labeled A, B, C, and the sides opposite each vertex labeled a, b, and c.


The Law of Cosines is useful when you are dealing with three sides and one angle; if you know three of those values, you can use the formula to figure out the fourth value.
If you’re trying to determine one of the side lengths, use this version.
If you’re trying to determine an angle measure, use this version.
In either case, the side you name c must be opposite the angle you name C.
Exercises
- Amateur surveyors have determined that the distance from
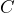 to
to  is 53 meters, the distance from
is 53 meters, the distance from 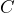 to
to 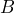 is 75 meters, and the measure of
is 75 meters, and the measure of 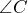 is
is  , as shown in the figure below. What is the length of the pond?
, as shown in the figure below. What is the length of the pond?

- Determine the measure of each angle.