Radian Measure

Morgan Chase

34 Radian Measure

You may use a calculator as needed in this module.

In everyday life, we measure angles in degrees. However, degrees are an arbitrary unit of measure; why is a right angle 90 degrees and a full circle 360 degrees?^[1]

Another unit that is used in surveying is the gradian; a right angle is equal to 100 gradians. Using gradians makes some calculations easier, but this is still an arbitrary unit of measure.

In this module, we will focus on a third unit of angle measure known as radians.

Radian Measure

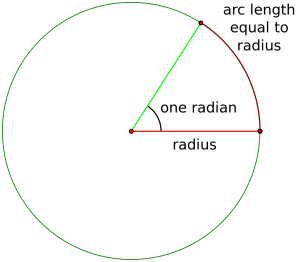
angle labeled 1 radian with arc length equal to radius — Image by Adrignola on Wikimedia Commons.

To explain radian measure, we can visualize a circle. Next, let’s measure a length along the circumference of a circle that is equal to the radius. Finally, let’s draw an angle with its vertex at the center of the circle, intersecting the circle at the endpoints of this arc. The measure of this angle is defined to be 1 radian.

In fewer words, a radian is the measure of the central angle that forms an arc equal to the circle’s radius.

Because the circumference of a circle is $2\pi$ times the radius, there are $2\pi$ radians in one full circle, and therefore $2\pi$ radians are equivalent to $360^\circ$ .