Height (Length)

Height is a common body measurement typically measured in feet (ft) + inches (in) in the United States and centimeters (cm) elsewhere. These are length measurements, so the SI unit would be meters. Keep in mind that x feet + y inches is commonly denoted as x‘ y“.

Range of Motion

Range of motion is a common body measurement, especially while diagnosing injury or disease, tracking progress during physical therapy, or working to improve flexibility or form. Range of motion is often defined as an angle measured in degrees (°)through which a joint moves away from a reference position as seen in this video demonstration of how to use a goniometer for range of motion measurement.

For example, in the image below the joint angle is 60° and the reference position is a nearly horizontal forearm creating a 90° between the bicep muscle and the bones of the forearm. (This is not necessarily how the reference angle is defined for measuring elbow range of motion by health professionals)

^[1]

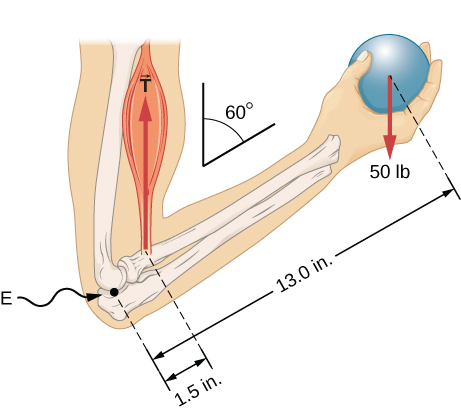
At times we might want to know how far something moves as it travels through an angle. For example, as the arm in the image below moved from 90° to 60° what distance did the hand and ball cover? In such cases, and many others, working with angles in radians (rads) can be helpful. We can convert between degrees and radians using the conversion factor:

(1)  

For example, in moving from 90° to the 60° seen in the previous diagram, the lower arm traversed 30° so we can convert this to radians:

The distance we are trying to calculate is known as the arc length, and to find it we multiply the angle in units of radians by the distance from the rotation point (also known as the radius).  The symbols commonly used for arc length, angle and radius are: , , and so in our example we will have:

We have found that the hand moves a distance of 6.8 inches when the forearm moves from horizontal to 60° of flexion. We have also discovered that rads are a placeholder unit, meaning that when rads gets multiplied by another unit, rads doesn’t necessarily show up  in the final units. Finally, we realize that we need to start keeping track of which symbols are most commonly used for which quantities. The following table shows the symbols most often used for some of the common quantities that we will encounter in this textbook. As we introduce new quantities going forward we will provide the common symbol in parenthesis when the quantity is first introduced.

Table of Common Physical Quantities and their Typical Symbols

Quantity	Most Commonly Used Symbol
Time	t
Length/width/distance/height/radius	l/w/d/h/r
Speed	v
Acceleration	a
Angle	θ
Area	A
Volume	V
Mass	m
Density	ρ
Force	F
Torque
Work	W
Mechanical Energy/Internal Energy/Heat	E/U/Q
Power	P
Momentum
Temperature	T
Entropy	S